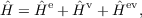
At the effective single-particle level, the Hamiltonian of the coupled system of electrons and vibrations is given by [181]
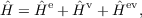 | (15.1) |
where the first term Ĥe describes the electronic system and the second term Ĥv the vibrational degrees of freedom. The last term in the Hamiltonian
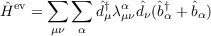 | (15.2) |
describes the first order electron-vibration (EV) interaction. The EV coupling constants are given as
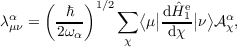 | (15.3) |
where χ = (k,u) is a shorthand notation that refers both to the displacement of atom k from the
equilibrium value of the position 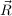 k along the Cartesian component Rk,u with u = x,y,z as well
as the index pair itself. Furthermore,
k along the Cartesian component Rk,u with u = x,y,z as well
as the index pair itself. Furthermore, 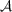 χα =
χα =  χα∕
χα∕ are the mass-normalized normal modes,
obtained from the eigenvectors
are the mass-normalized normal modes,
obtained from the eigenvectors  χα of the dynamical matrix as calculated from the aoforce
module [181].
χα of the dynamical matrix as calculated from the aoforce
module [181].